Runge's theorem
In complex analysis, Runge's theorem, also known as Runge's approximation theorem, named after the German Mathematician Carl Runge, and put forward by him in the year 1885, states the following: If K is a compact subset of C (the set of complex numbers), A is a set containing at least one complex number from every bounded connected component of C\K, and f is a holomorphic function on an open set containing K, then there exists a sequence 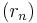 of rational functions all of whose poles are in A such that the sequence
of rational functions all of whose poles are in A such that the sequence 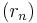 approaches the function f uniformly on K.
approaches the function f uniformly on K.
Note that not every complex number in A need be a pole of every rational function of the sequence 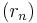 . We merely know that if some
. We merely know that if some 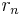 of the sequence has poles, those poles are in A.
of the sequence has poles, those poles are in A.
One of the things that makes this theorem so powerful is that one can choose the set A at will. In other words, one can pick any complex numbers as one wishes from the bounded connected components of C\K. Then the theorem guarantees the existence of a sequence of rational functions with poles only in those chosen numbers.
In the special case that C\K is a connected set, the set A in the theorem will clearly be empty. And since rational functions with no poles are indeed nothing but polynomials, we get the following corollary: If K is a compact subset of C such that C\K is a connected set, and f is a holomorphic function on K, then there exists a sequence of polynomials 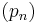 that approaches f uniformly on K.
that approaches f uniformly on K.
A slightly more general version of this theorem is obtained if one takes A to be a subset of the Riemann sphere C∪{∞} and then requires A to intersect also the unbounded connected component of K (which now contains ∞). That is, in the formulation given above, the rational functions may turn out to have a pole at infinity, while in the more general formulation the pole can be chosen instead anywhere in the unbounded connected component of K.
See also
References
- John B. Conway, A Course in Functional Analysis, Springer; 2 edition (1997), ISBN 0-387-97245-5.
- Robert E. Greene and Steven G. Krantz, Function Theory of One Complex Variable, American Mathematical Society; Second Edition (2002), ISBN 0-8218-2905-X.